[Back to the Solutions Menu]
Quiz: Circle Module
Instructions:Answer all the following questions in the space provided. Simplify all answers.
  1. Identify the centre and radius of the circle defined by:

    a) x2 + y2 = 1 centre (0, 0) radius 1

    b) (x - 3)2 + (y + 4)2 = 24 centre (3, -4) radius Ö24

    c) x2 + (y - 7)2 = 121 centre (0, 7) radius 11


  2. A circle centred at the origin has a radius of (Ö5)/ 2. = 1.12 (approximately)

    a) Sketch the graph of this circle on the graph paper provided.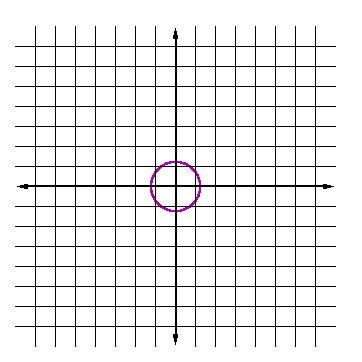














    b) Write the equation of this circle in standard form.x2 + y2 = 5/4

    c) Write the equation of this circle in general form. 4x2 + 4y2 - 5 = 0


  3. A circle centred at (-7, -5/2) has a radius of 5.
    a) Sketch the graph of this circle on the graph paper provided.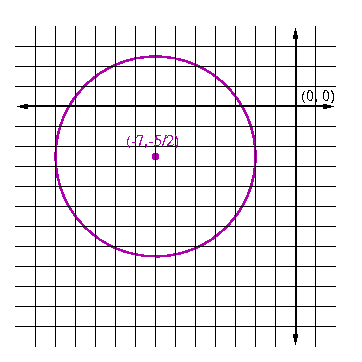














    b) Write the equation of this circle in standard form.
    (x + 7)2 + (y + 5/2)2 = 25

    c) Write the equation of this circle in general form.
    x2 + y2 + 14x + 5y + 121/4 = 0
    or 4x2 + 4y2 + 56x + 20y + 121 = 0


  4. Describe the effect that varying h, k and r in the standard equation (x - h)2 + (y - k)2 = r2 has on the graph of a circle by completing the following chart.

    The Effect of h, k and r on the graph of (x - h)2 + (y - k)2 = r2.
    Variable The value of the variable decreases The value of the variable increases The value of the variable is 0.
    h
    		 
    circle shifts left
          		 
    circle shifts right
         		  
    circle is centred somewhere along the y-axis
     
    k
    		 
    circle shifts down
          		 
    circle shifts up
         		  
    circle is centred somewhere along the x-axis
     
    |r|
    		 
    circle gets smaller
          		 
    circle gets larger
          		 
    result is a point
     


  5. The equation x2 + y2 - 8x + 20y + 67 = 0 defines a circle.
    a) Determine the centre and radius of this circle.

    Centre:
    (4, -10)

    Radius:

    7

    b) Sketch a picture of the circle on the provided graph paper.

  6. What must be true of the distances between 0 and each point A, B and C, if A, B and C lie on a circle whose centre is (0, 0)?

    If A, B and C all lie on the same circle centred at the origin, then the distances from each of A, B and C to the origin must be equal.


  7. If the equation Ax2 + By2 + Cx + Dy + F = 0 defines a circle, then what must be true about the values of the coefficients A and B?

    A must equal B

  8. A circle is formed when a horizontal plane cuts a double-napped cone.
    Describe what happens to the circle as the horizontal plane:

    a) moves closer to the vertex
    the circle gets smaller
    b) moves away from the vertex
    the circle gets bigger
    c) cuts the double-napped cone at the vertex
    the result is a point


  9. The following circle is centred at (0, 0).

    a) What is the radius of this circle? Radius =  6

    b) Write the standard equation of this circle.    x2 + y2 = 36

    c) If the radius of the circle remains constant, then what would be the standard equation of the circle centred at the point A = (4, -6)?

    (x - 4)2 + (y + 6)2 = 36

    d) Sketch the translated circle in the above graph.

    e) Explain how the circle was translated.

    The circle was translated right 4 units and down 6 units



  10. A circle is defined by the standard equation (x + 4)2 + (y + 6)2 = 169 and x2 + y2 + 8x + 12y - 117 = 0.
    a) Show that these two equations are equivalent.

    Let's expand the first equation:
    x2 + 8x + 16 + y2 + 12y + 36 - 169 = 0
    ==> x2 + y2 + 8x + 12y + (16 + 36 - 169) = 0
    ==> x2 + y2 + 8x + 12y - 117 = 0

    b) When two equations are equivalent they have identical solution sets. Verify that the point (-9, 6) is a solution of both equations.

    Substitute     (-9, 6) into both equations:
    (-9 + 4)2 + (6 + 6)2
    = -52 + 122
    = 25 + 144
    = 169    		 (-9)2 + (6)2 + 8(-9) + 12(6) - 117
    = 81 + 36 - 72 + 72 - 117
    = 0
     
  11. In the form x2 + y2 = P, a circle exists when P > 0.
    In the form x2 + y2 + F = 0, a circle exists when F < 0.
    Show how these two statements are saying the same thing.

    x2 + y2 + F = 0, can be rewritten as x2 + y2 = -F, which implies that P = -F. Therefore if P > 0 in the equation x2 + y2 = P, then -F > 0 in the equation x2 + y2 = -F, which implies F < 0 in the equation x2 + y2 + F = 0.


  12. Explain or use the provided diagrams to show how either the distance formula or the Pythagorean Theorem can be used to:
    a) Derive the standard equation of a circle centred at (0, 0).
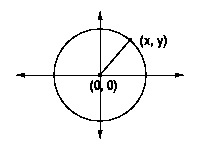    Let r be the radius of this circle. Therefore r is the distance between the point (x, y) and the origin.
    Applying the distance formula yields the equation [x2 + y2]½ = r
    Squaring both sides yields the standard equation we are looking for, x2 + y2 = r2.


    b) Derive the standard equation of a circle centred at (h, k).
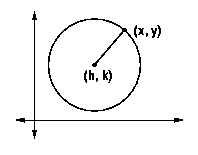    Let r be the radius of this circle. Therefore, r is the distance between the point (x, y) and the centre point (h, k). Applying the distance formula yields the equation [(x-h)2 + (y-k)2]½ = r.
    Squaring both sides yeilds the standard equation for circles centred at (h, k), (x-h)2 + (y-k)2 = r2