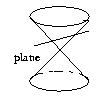Instructions:Answer all the following questions in the space provided. Simplify all answers.
- For the following standard equations, identify the centre of the ellipse and the lengths of the horizontal and vertical axes.
a) x2/ 16 + y2/ 49 = 1
| Centre: (0, 0)
Horizontal Axis: 8
Vertical Axis: 14
b) (x - 1)2/ 4 + y2/ 25 = 1
|
Centre: (1, 0)
Horizontal Axis: 4
Vertical Axis: 10
c) (x - 10)2/ 20 + (y + 7)2/ 144 = 1
|
Centre: (10, -7)
Horizontal Axis: 2Ö20
Vertical Axis: 24
| | |
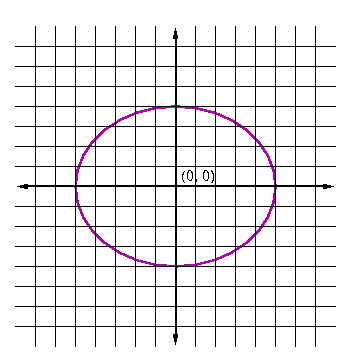
- An ellipse centred at the origin has a horizontal axis of length 10 and a vertical axis of length 8.
a) Sketch the graph of this ellipse on the graph paper provided.
|  |
| |
| b) Write the equation of this ellipse in standard form. | x2/ 25 + y2/ 16 = 1 |
| |
| c) Write the equation of this ellipse in general form. | 16x2 + 25y2 - 400 = 0 |
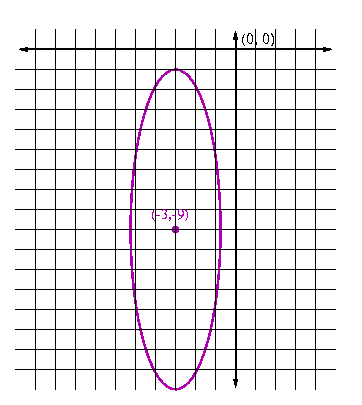
- An ellipse centred at (-3, -9) has a horizontal axis of length 2Ö5 and a vertical axis of length 16.
a) Sketch the graph of this ellipse on the graph paper provided.
|  |
| |
| b) Write the equation of this ellipse in standard form. |
(x + 3)2/ 5 + (y + 9)2/ 64 = 1 |
| |
| c) Write the equation of this ellipse in general form. | 64x2 + 5y2 + 384x + 90y + 781 = 0 |
- Describe the effect that varying h and k in the standard equation (x - h)2/ a2 + (y - k)2/ b2 = 1 has on the graph of an ellipse by completing the following chart.
The Effect of h, k and r on the graph of (x - h)2/ a2 + (y - k)2/ b2 = 1.
| Variable | The value of the variable decreases | The value of the variable increases | The value of the variable is 0. |
|---|
| h |
ellipse shifts down
|
ellipse shifts up
|
ellipse is centred along the y-axis
|
| k |
ellipse shifts left
|
ellipse shifts right
|
ellipse is centred along the x-axis
|
- Describe the effect that varying a and b in the standard equation (x - h)2/ a2 + (y - k)2/ b2 = 1 has on the graph of an ellipse by completing the following chart.
The Effect of a and b on the graph of (x - h)2/ a2 + (y - k)2/ b2 = 1
| Value of a and b |
Effect on the graph of the ellipse |
|---|
| a > b |
horizontal axis is longer than the vertical axis
|
| a < b |
vertical axis is longer than the horizontal axis
|
| a = b |
the ellipse is a special ellipse called a circle
|
- The equation 16x2 + y2 - 160x - 16y + 400 = 0 defines an ellipse.
a) Determine the centre of the ellipse and the lengths of its horizontal and vertical axes.
Centre: |
(5, 8) |
Horizontal axis length: |
4
Vertical axis length: | |
16
|
b) Sketch a picture of this ellipse on the provided graph paper.

- An ellipse is defined by the standard equation (x + 9)2/ 9 + (y + 4)2/ 36 = 1 and by the general equation 4x2 + y2 + 72x + 8y + 304 = 0.
a) Show that these two equations are equivalent.
Clear the denominators in the first equation:
4(x + 9)2 + (y + 4)2 = 36
Expand and simplify: 4(x2 + 18x + 81) + (y2 + 8y + 16) - 36 = 0
==>4x2 + 72x + 324 + y2 + 8y + 16 - 36 = 0
==>4x2 + y2 + 72x + 8y + 304 = 0
b) When two equations are equivalent they have identical solution sets. Verify that the point (-12, -4) is a solution of both equations.
Substitute (-12, -4) into both equations:
(x + 9)2/ 9 + (y + 4)2/ 36 = 1
= (-12 + 9)2/ 9 + (-4 + 4)2/ 36
= (-3)2/ 9 + 0
= 9/ 9
= 1 |
4x2 + y2 + 72x + 8y + 304 = 0
= 4(-12)2 + (-4)2 + 72(-12) + 8(-4) + 304
= 4(144) + 16 - 864 - 32 + 304
= 576 + 16 - 864 - 32 + 304
= 0 |
- If the equation Ax2 + By2 + Cx + Dy + F = 0 defines an ellipse, then what must be true about the values of the coefficients A and B?
For the general equation to represent an ellipse, A and B must have the same sign. In order for it to represent an ellipse which is not a circle, A must not equal B.
- Explain why a circle is a special ellipse.
The standard circle equation is (x - h)2 + (y - k)2 = r2, which can be rewritten as (x - h)2/ r2 + (y - k)2/ r2 = 1, which is the standard ellipse equation. A circle is just an ellipse where a = b.
- An ellipse is formed when a double-napped cone is cut by a plane that cuts exactly one nap of the cone. Describe what happens to the ellipse as:
a) The plane gradually tilts towards being parallel to the generator of the double-napped cone.
the lengths of the horizontal and vertical axes of the ellipse become more different; the ellispe elongates
|
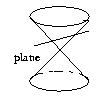 |
b) The plane gradually tilts towards being perpendicular to the vertical axis of the double-napped cone.
the lengths of the axes of the horizontal and vertical axes become more similar; the ellipse becomes more circlular |
c) The plane cuts the cone exactly perpendicular to the vertical axis.
the result is the special ellipse called a circle |
- The following ellipse is centred at (0, 0).

| a) What are the lengths of the horizontal and vertical axes? |
horizontal |
12 units |
vertical |
18 units |
b) Write the standard equation of this ellipse. |
x2/ 36 + y2/ 81 = 1 |
c) If the lengths of the axes remain the same, what would be the standard equation of this ellipse centred at the point P? |
(x + 7)2/ 36 + (y - 2)2/ 81 = 1 |
d) Sketch the graph of the translated ellipse in the above graph paper.
e) Describe how the ellipse was translated.
The ellipse was translated 7 units left and 2 units up.
|
|
|