Instructions:Answer all the following questions in the space provided. Simplify all answers.
- Which conic section is defined by the following standard equations.
a) x2 + y2 = 49 circle
b) (x - 4)2/25 - (y + 2)2/20 = 1 hyperbola
c) x = 10(y - 8)2 parabola
d) x2/4 + (y - 5)2/16 = 1
ellipse
- Draw pictures that shows the three different ways that a line and a circle can intersect.
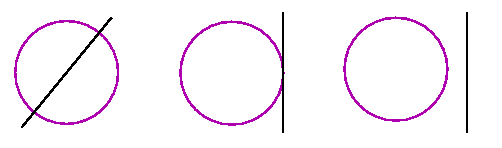
- Two quadratic equations can intersect 0, 1, 2, 3 or 4 times. Draw a picture that illustrates:
a) A hyperbola and circle intersecting once
|
b) A parabola and an ellipse intersecting twice.
|
c) A parabola and circle intersecting at 3 points
|
d) A circle and an ellipse intersecting at 4 points.
|
- The following questions involve the equation 8x2 + 5y2 - 64x + 20y + 108 = 0.
a) Inspect the coefficients in the equation. State which conic section this equation might represent and explain your answer.
This equation looks like it should represent an ellipse because A = +8 and B = +5, so A and B have the same sign.
(Since A does not equal B, we know this isn't a circle.)
b) Rewrite the equation in standard form to show that your answer in the previous question is correct.
8x2 + 5y2 - 64x + 20y + 108 = 0.
8(x2 - 8x) + 5(y2 + 4y) + 108 = 0
8(x2 - 8x + 16) - 8(16) + 5(y2 + 4y + 4) - 5(4) + 108 = 0
8(x - 4)2 + 5(y + 2)2 = -108 + 128 + 20 = 40
8(x - 4)2/ 40 + 5(y + 2)2/ 40 = 1
(x - 4)2/ 5 + (y + 2)2/ 8 = 1
Since this is the standard equation for an ellipse centred at (4, -2) with axes of lengths 2Ö5 and 2Ö8, we see that our prediction was correct.
- The following questions involve the equation x2 + 12x - 3y + 39 = 0.
a) Inspect the coefficients in the equation. State which conic section this equation might represent and explain your answer.
This equation looks like it should represent a parabola that opens up or down, because there is an x2 term, but no y2 term.
b) Rewrite the equation in standard form to show that your answer in the previous question is correct.
x2 + 12x - 3y + 39 = 0
3y = x2 + 12x + 39
3y = (x2 + 12x + 36) - 36 + 39
y = 1/3[(x + 6)2 + 3]
y = 1/3(x + 6)2 + 1
This is the standard form of a parbola that opens up and with its vertex at (-6, 1). Therefore, our prediction was correct.
- A linear-quadratic system of equation consists of the equations:
y + (3/4)x = 0 and
x2 + y2 - 25 = 0.
a) Sketch the graph of the system on the provided graph
paper.

b) From the graph, estimate the solution of the system.
Approximate points of intersection are (-4, 3) and (4, 3).
c) Use algebra to find the exact solutions to the system.
Solve the first equation for y: y = -(3/4)x
Substitute this expression into the second equation: x2 + (3/4x)2 - 25 = 0
x2 + 9x2/ 16 - 25 = 0
16x2 + 9x2 - 400 = 0
25x2 = 400
x2 = 16
x = ±4
Substitute x = 4 and x = -4 back into the first equation:
y = -(3/4)(4) = -3 and y = -(3/4)(-4) = 3.
So the points of intersection are (4, -3) and (-4, 3).
d) Show how to check your answer in part (c) by substituting the solutions back into the original equations.
Substitute both points into the second equation:
x2 + y2 - 25 = (4)2 + (-3)2 = 16 + 9 = 25
x2 + y2 - 25 = (-4)2 + (3)2 = 16 + 9 = 25
- The equations x2 + 4y2 - 36 = 0 and x - 2y - 6 = 0 define a linear-quadratic system of equations.
a) Sketch the graph of the system on the provided graph paper.

b) From the graph, estimate the solution of the system.
The points of intersection are approzimatley (0, -3) and (6, 0).
c) Use algebra to find the exact solutions to the system.
Solve the second equation for x: x = 2y + 6
Substitute this expression for x into the first equation: (2y + 6)2 + 4y2 - 36 = 0
4y2 + 24y + 36 + 4y2 - 36 = 0
8y2 + 24y = 0
8y(y + 3) = 0
y = 0 or y = -3
Substitute these points back into the second equation:
x = 2y - 6 = 2(0) + 6 = 6 and x = 2(-3) + 6 = -6 + 6 = 0
So the points of intersection are (6, 0) and (0, -3).
d) Show how to check your answer in part (c) by substituting the solutions back into the original equations.
Substitute both points back into the first equation:
x2 + 4y2 - 36 = (6)2 + 4(0)2 - 36 = 36 - 36 = 0
x2 + 4y2 - 36 = (0)2 + 4(-3)2 - 36 = 4(9) - 36 = 36 - 36 = 0
- A quadratic-quadratic system of equations consists of the following:
4x2 - y2 - 7 = 0 and
2x2 + 5y2 - 9 = 0.
a) Sketch the graph of the system on the provided graph paper.
b) From the graph, estimate the solution of the system.
The points of intersection are approximately (1.4, 1), (1.4, -1), (-1.4, 1) and (-1.4, -1).
c) Use algebra to find the exact solutions to the system.
Multiply the second equation by 2 and subtract it from the first equation:
4x2 - y2 - 7 = 0
- (4x2 + 10y2 - 18 = 0)
-11y2 + 11 = 0
So y2 = 1
y = ± 1
Substitute y = 1 into the first equation:
4x2 - (1)2 - 7 = 0
x2 = 8/4 = 2
x = ± Ö2 = ± 1.414
So two of the points of intersection are (+Ö2, 1) and (-Ö2, 1).
Similarily, substituting y = -1 into the first equation gives us the other two solutions, (+Ö2, -1) and (-Ö2, -1)
d) Show how to check your answer in part (c) by substituting the solutions back into the original equations.
We will just check the point (+Ö2, 1) in the second equation.
2(Ö2)2 + 5(1)2 - 9
= 2(2) + 5(1) - 9
= 4 + 5 - 9
= 0
- The equations x2 + y2 - 12 = 0 and x2 - 4y = 0 define a quadratic-quadratic system of equations.
a) Sketch the graph of the system on the provided graph paper.
b) From the graph, estimate the solution of the system.
The points of intersection are approximately (2.8, 2) and (-2.8, 2).
c) Use algebra to find the exact solutions to the system.
Subtract the second equation from the first:
x2 + y2 - 12 = 0
- (x2 - 4y = 0)
y2 + 4y - 12 = 0
Solve for y:
(y - 2)(y + 6) = 0
y = 2 and y = -6
If we put y = -6 into the second equation we get:
x2 - 4(-6) = 0
==> x2 = -24
This has no real solutions, so we can discard y = -6 from our list of possible solutions.
Substitute y = 2 into the second equation:
x2 - 4(2) = 0
==> x2 = 8
x = ± Ö8 = ± 2.828
So the two points of intersection are (Ö8, 2) and (-Ö8, 2).
d) Show how to check your answer in part (c) by substituting the solutions back into the original equations.
Substitute both points into the first equation:
x2 + y2 - 12 = (Ö8)2 + (2)2 - 12 = 8 + 4 - 12 = 0
x2 + y2 - 12 = (-Ö8)2 + (2)2 - 12 = 8 + 4 - 12 = 0
- In Figure 1, a circle is translated over the fixed graph of an ellipse by varying the value of h in the circle equation (x - h)2 + y2 = 4.
For what positive values of h does the circle intersect the ellipse at:
Figure 1

a) one point? h = 3 and h = 9
b) two points? 3 < h < 5 and 7 < h < 9
c) three points? h = 5 and h = 7
d) four points? 5 < h < 7
e) no points? h < 3 or h > 9
|



